こんにちは、リンス(@Lins016)です。
今回は方べきの定理の証明について学習していこう。
方べきの定理の証明(3パターン)

\(\small{ \ \mathrm{PT}^2=\mathrm{PM}\cdot\mathrm{PN} \ }\)

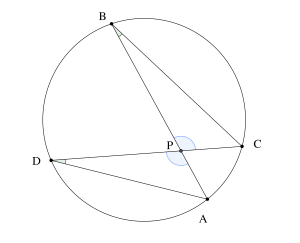
円の内部に点Pがある方べきの定理の証明
\(\small{ \ \triangle \mathrm{PAD} \ }\)と\(\small{ \ \triangle \mathrm{PCB} \ }\)について
\(\small{ \ \stackrel{ \Large \frown }{ \mathrm{AC} } \ }\)の円周角より
\(\small{ \ \angle\mathrm{PDA}=\angle\mathrm{PBC} \ }\)
対頂角より
\(\small{ \ \angle\mathrm{APD}=\angle\mathrm{CPB} \ }\)
\(\small{ \ 2 \ }\)つの角がそれぞれ等しいので
\(\small{ \ \triangle \mathrm{PAD} \backsim \triangle \mathrm{PCB} \ }\)
\(\small{ \ \mathrm{PA}:\mathrm{PC}=\mathrm{PD}:\mathrm{PB} \ }\)
\(\small{ \ \therefore \mathrm{PA}\cdot\mathrm{PB}=\mathrm{PC}\cdot\mathrm{PD} \ }\)

円の外部に点Pがある方べきの定理の証明
\(\small{ \ \triangle \mathrm{PAD} \ }\)と\(\small{ \ \triangle \mathrm{PCB} \ }\)について
\(\small{ \ \stackrel{ \Large \frown }{ \mathrm{AC} } \ }\)の円周角より
\(\small{ \ \angle\mathrm{ADC}=\angle\mathrm{ABC} \ }\)
\(\small{ \ \angle \mathrm{P} \ }\)は共通
\(\small{ \ 2 \ }\)つの角がそれぞれ等しいので
\(\small{ \ \triangle \mathrm{PAD} \backsim \triangle \mathrm{PCB} \ }\)
\(\small{ \ \mathrm{PA}:\mathrm{PC}=\mathrm{PD}:\mathrm{PB} \ }\)
\(\small{ \ \therefore \mathrm{PA}\cdot\mathrm{PB}=\mathrm{PC}\cdot\mathrm{PD} \ }\)

接線を含む方べきの定理の証明
\(\small{ \ \triangle \mathrm{PMT} \ }\)と\(\small{ \ \triangle \mathrm{PTN} \ }\)について
\(\small{ \ \mathrm{T} \ }\)は円の接線だから接弦定理より
\(\small{ \ \angle\mathrm{PTM}=\angle\mathrm{PNT} \ }\)
\(\small{ \ \angle \mathrm{P} \ }\)は共通
\(\small{ \ 2 \ }\)つの角がそれぞれ等しいので
\(\small{ \ \triangle \mathrm{PMT} \backsim \triangle \mathrm{PTN} \ }\)
\(\small{ \ \mathrm{PM}:\mathrm{PT}=\mathrm{PT}:\mathrm{PN} \ }\)
\(\small{ \ \therefore \mathrm{PT}^2=\mathrm{PM}\cdot\mathrm{PN} \ }\)


