こんにちは、リンス(@Lins016)です。
今回は正弦定理の証明について学習していこう。
\(\small{ \ \displaystyle \frac{a}{\sin \mathrm{A}}=\displaystyle \frac{b}{\sin \mathrm{B}}=\displaystyle \frac{c}{\sin \mathrm{C}}=2R \ }\)
\(\small{ \ R \ }\)は\(\small{ \ \triangle \mathrm{ABC} \ }\)の外接円の半径
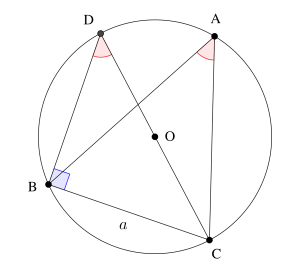
(1)\(\small{ \ \angle \mathrm{A} \ }\)が鋭角の場合
\(\small{ \ \stackrel{\Large \frown}{\mathrm{BC}} \ }\)の円周角より\(\small{ \ \angle \mathrm{A}=\angle \mathrm{D} \ }\)
よって\(\small{ \ \sin \mathrm{A}=\sin \mathrm{D} \ }\)
\(\small{ \ \mathrm{CD} \ }\)は外接円の直径より
\(\small{ \ \angle \mathrm{DBC}=90^{ \circ } \ }\)
\(\small{ \ \displaystyle \frac{\mathrm{BC}}{\mathrm{CD}}=\sin \mathrm{D} \ }\)
\(\small{ \ \therefore \displaystyle \frac{a}{2R}=\sin \mathrm{A} \ }\)
よって\(\small{ \ \displaystyle \frac{a}{\sin \mathrm{A}}=2R \ }\)
同様に
\(\small{ \ \displaystyle \frac{b}{\sin \mathrm{B}}=2R, \ \ \displaystyle \frac{c}{\sin \mathrm{C}}=2R \ }\)
よって
\(\small{ \ \displaystyle \frac{a}{\sin \mathrm{A}}=\displaystyle \frac{b}{\sin \mathrm{B}}=\displaystyle \frac{c}{\sin \mathrm{C}}=2R \ }\)
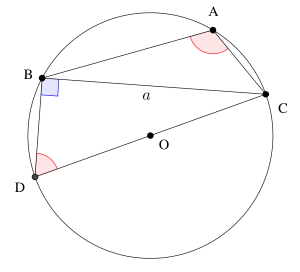
(2)\(\small{ \ \angle \mathrm{A} \ }\)が鈍角の場合
各点は円周上にあるので対角の和は\(\small{ \ 180^{\circ} \ }\)になる。
\(\small{ \ \therefore \angle \mathrm{A}+\angle \mathrm{D}=180^{\circ} \ }\)
よって
\(\small{ \ \mathrm{CD} \ }\)は外接円の直径より
\(\small{ \ \angle \mathrm{DBC}=90^{ \circ } \ }\)
\(\small{ \ \displaystyle \frac{\mathrm{BC}}{\mathrm{CD}}=\sin \mathrm{D} \ }\)
\(\small{ \ \sin \mathrm{D}=\sin \left(180^{\circ}-\mathrm{A}\right)=\sin \mathrm{A} \ }\)
\(\small{ \ \therefore \displaystyle \frac{a}{2R}=\sin \mathrm{A} \ }\)
よって\(\small{ \ \displaystyle \frac{a}{\sin \mathrm{A}}=2R \ }\)
\(\small{ \ \angle \mathrm{B} \ }\)、\(\small{ \ \angle \mathrm{C} \ }\)は鋭角より(1)を利用して
\(\small{ \ \displaystyle \frac{b}{\sin \mathrm{B}}=2R, \ \ \displaystyle \frac{c}{\sin \mathrm{C}}=2R \ }\)
よって
\(\small{ \ \displaystyle \frac{a}{\sin \mathrm{A}}=\displaystyle \frac{b}{\sin \mathrm{B}}=\displaystyle \frac{c}{\sin \mathrm{C}}=2R \ }\)