こんにちは、リンス(@Lins016)です。
今回は加法定理と15度と75度の三角比(三角関数)について学習していこう。
加法定理の利用
三角関数は公式が多い単元で覚えるのがすごく大変だと思うけど、最低でも有名角の三角関数の値と加法定理は覚えておかないといけない。これを覚えてなかったら0点だっていうぐらい重要だからね。
まずは確実に加法定理を覚えよう。
・加法定理
\(\small{ \ \sin\left(\alpha+\beta\right)=\sin\alpha\cos\beta+\cos\alpha\sin\beta \ }\)
\(\small{ \ \sin\left(\alpha-\beta\right)=\sin\alpha\cos\beta-\cos\alpha\sin\beta \ }\)
\(\small{ \ \cos\left(\alpha+\beta\right)=\cos\alpha\cos\beta-\sin\alpha\sin\beta \ }\)
\(\small{ \ \cos\left(\alpha-\beta\right)=\cos\alpha\cos\beta+\sin\alpha\sin\beta \ }\)
\(\small{ \ \tan\left(\alpha+\beta\right)=\displaystyle\frac{\tan\alpha+\tan\beta}{1-\tan\alpha\tan\beta} \ }\)
\(\small{ \ \tan\left(\alpha-\beta\right)=\displaystyle\frac{\tan\alpha-\tan\beta}{1+\tan\alpha\tan\beta} \ }\)
・15度\(\small{ \ \left(\displaystyle\frac{\pi}{12}\mathrm{rad}\right) \ }\)の三角比
\(\small{ \ \sin\displaystyle\frac{\pi}{12}=\displaystyle\frac{\sqrt{6}-\sqrt{2}}{4} \ }\)
\(\small{ \ \cos\displaystyle\frac{\pi}{12}=\displaystyle\frac{\sqrt{6}+\sqrt{2}}{4} \ }\)
\(\small{ \ \tan\displaystyle\frac{\pi}{12}=2-\sqrt{3} \ }\)
・75度\(\small{ \ \left(\displaystyle\frac{5}{12}\pi\mathrm{rad}\right) \ }\)の三角比
\(\small{ \ \sin\displaystyle\frac{5}{12}\pi=\displaystyle\frac{\sqrt{6}+\sqrt{2}}{4} \ }\)
\(\small{ \ \cos\displaystyle\frac{5}{12}\pi=\displaystyle\frac{\sqrt{6}-\sqrt{2}}{4} \ }\)
\(\small{ \ \tan\displaystyle\frac{5}{12}\pi=2+\sqrt{3} \ }\)
加法定理
\(\small{ \ \sin\displaystyle\frac{\pi}{6}=\displaystyle\frac{1}{2} \ }\)、\(\small{ \ \sin\displaystyle\frac{\pi}{3}=\displaystyle\frac{\sqrt{3}}{2} \ }\)、\(\small{ \ \sin\displaystyle\frac{\pi}{2}=1 \ }\)だから\(\small{ \ \sin\left(\alpha+\beta\right)=\sin\alpha+\sin\beta \ }\)が成り立たないのがわかるよね。
\(\small{ \ \sin\left(\alpha+\beta\right)=\sin\alpha+\sin\beta \ }\)ってしたくなるけど、成り立たないから絶対にしたらだめだよ。加法定理があることを忘れてこの式を使ってる人よく見かけるからね。
この\(\small{ \ \sin\left(\alpha+\beta\right) \ }\)を求める式こそ加法定理っていうんだ。加法定理は三角関数の中でもかなり重要な式になるから確実に覚えよう。
\(\small{ \ \sin\left(\alpha+\beta\right)=\sin\alpha\cos\beta+\cos\alpha\sin\beta \ }\)
\(\small{ \ \sin\left(\alpha-\beta\right)=\sin\alpha\cos\beta-\cos\alpha\sin\beta \ }\)
\(\small{ \ \cos\left(\alpha+\beta\right)=\cos\alpha\cos\beta-\sin\alpha\sin\beta \ }\)
\(\small{ \ \cos\left(\alpha-\beta\right)=\cos\alpha\cos\beta+\sin\alpha\sin\beta \ }\)
\(\small{ \ \tan\left(\alpha+\beta\right)=\displaystyle\frac{\tan\alpha+\tan\beta}{1-\tan\alpha\tan\beta} \ }\)
\(\small{ \ \tan\left(\alpha-\beta\right)=\displaystyle\frac{\tan\alpha-\tan\beta}{1+\tan\alpha\tan\beta} \ }\)
覚えるのすごく大変って人はこのあたりをチェックして、自分の覚えやすい語呂合わせを見つけてみてもいいかもね。
難関大学を受験する人は加法定理の証明も頭に入れておこう。
15度と75度の三角比
数学Ⅱでは弧度法を利用するから\(\small{ \ 15 \ }\)度は\(\small{ \ \displaystyle\frac{\pi}{12}\mathrm{rad} \ }\)、\(\small{ \ 75 \ }\)度は\(\small{ \ \displaystyle\frac{5}{12}\pi\mathrm{rad} \ }\)になるけど、加法定理を利用することで、この角度の三角比も求めることができるんだ。
\(\small{ \ 15 \ }\)度\(\small{=45 \ }\)度\(\small{-30 \ }\)度を利用して考えると
\(\small{\begin{eqnarray} \ \sin \displaystyle\frac{\pi}{12}&=&\sin\left(\displaystyle\frac{\pi}{4}-\displaystyle\frac{\pi}{6}\right)\\
&=&\sin\displaystyle\frac{\pi}{4}\cos\displaystyle\frac{\pi}{6}-\cos\displaystyle\frac{\pi}{4}\sin\displaystyle\frac{\pi}{6}\\
&=&\displaystyle\frac{\sqrt{6}-\sqrt{2}}{4} \ \end{eqnarray}}\)
\(\small{\begin{eqnarray} \ \cos \displaystyle\frac{\pi}{12}&=&\cos\left(\displaystyle\frac{\pi}{4}-\displaystyle\frac{\pi}{6}\right)\\
&=&\cos\displaystyle\frac{\pi}{4}\cos\displaystyle\frac{\pi}{6}+\sin\displaystyle\frac{\pi}{4}\sin\displaystyle\frac{\pi}{6}\\
&=&\displaystyle\frac{\sqrt{6}+\sqrt{2}}{4} \ \end{eqnarray}}\)
\(\small{\begin{eqnarray} \ \tan \displaystyle\frac{\pi}{12}&=&\displaystyle\frac{\sin \displaystyle\frac{\pi}{12}}{\cos \displaystyle\frac{\pi}{12}}\\
&=&2-\sqrt{3} \ \end{eqnarray}}\)
同様に\(\small{ \ 75 \ }\)度\(\small{=45 \ }\)度\(\small{+30 \ }\)度を利用して考えると
\(\small{\begin{eqnarray} \ \sin \displaystyle\frac{5}{12}\pi&=&\sin\left(\displaystyle\frac{\pi}{4}+\displaystyle\frac{\pi}{6}\right)\\
&=&\sin\displaystyle\frac{\pi}{4}\cos\displaystyle\frac{\pi}{6}+\cos\displaystyle\frac{\pi}{4}\sin\displaystyle\frac{\pi}{6}\\
&=&\displaystyle\frac{\sqrt{6}+\sqrt{2}}{4} \ \end{eqnarray}}\)
\(\small{\begin{eqnarray} \ \cos \displaystyle\frac{5}{12}\pi&=&\cos\left(\displaystyle\frac{\pi}{4}+\displaystyle\frac{\pi}{6}\right)\\
&=&\cos\displaystyle\frac{\pi}{4}\cos\displaystyle\frac{\pi}{6}-\sin\displaystyle\frac{\pi}{4}\sin\displaystyle\frac{\pi}{6}\\
&=&\displaystyle\frac{\sqrt{6}-\sqrt{2}}{4} \ \end{eqnarray}}\)
\(\small{\begin{eqnarray} \ \tan \displaystyle\frac{5}{12}\pi&=&\displaystyle\frac{\sin \displaystyle\frac{\pi}{12}}{\cos \displaystyle\frac{\pi}{12}}\\
&=&2-\sqrt{3} \ \end{eqnarray}}\)
このように加法定理を利用することで有名角以外の三角関数の値を求めることができるんだ。
15度間隔での三角関数
加法定理を利用することで\(\small{ \ 15 \ }\)度と\(\small{ \ 75 \ }\)度の三角関数の値がわかったわけだけど、この値を利用することで\(\small{ \ 15 \ }\)度間隔、つまり弧度法で\(\small{ \ \displaystyle\frac{\pi}{12} \ }\)の整数倍の三角関数の値が言えるんだ。
図の三角形を単位円に当てはめれば、その\(\small{ \ x \ }\)座標が\(\small{ \ \cos\theta \ }\)の値になるし、\(\small{ \ y \ }\)座標が\(\small{ \ \sin\theta \ }\)の値になるからね。
今まで覚えた有名角の\(\small{ \ \sin\theta \ }\)と\(\small{ \ \cos\theta \ }\)の値は\(\small{ \ 0, \ \displaystyle\frac{1}{2}, \ \displaystyle\frac{\sqrt{2}}{2}, \ \displaystyle\frac{\sqrt{3}}{2}, \ 1 \ }\)に\(\small{ \ \pm \ }\)を付けた値だけだったけど、これに\(\small{ \ \displaystyle\frac{\sqrt{6}+\sqrt{2}}{4} \ }\)と\(\small{ \ \displaystyle\frac{\sqrt{6}-\sqrt{2}}{4} \ }\)に\(\small{ \ \pm \ }\)を付けた値も加えることで\(\small{ \ 15 \ }\)度間隔の三角関数の値が言えることになる。
もちろん加法定理を利用して\(\small{ \ 15 \ }\)度の整数倍の三角関数の値を求めることもできるからね。
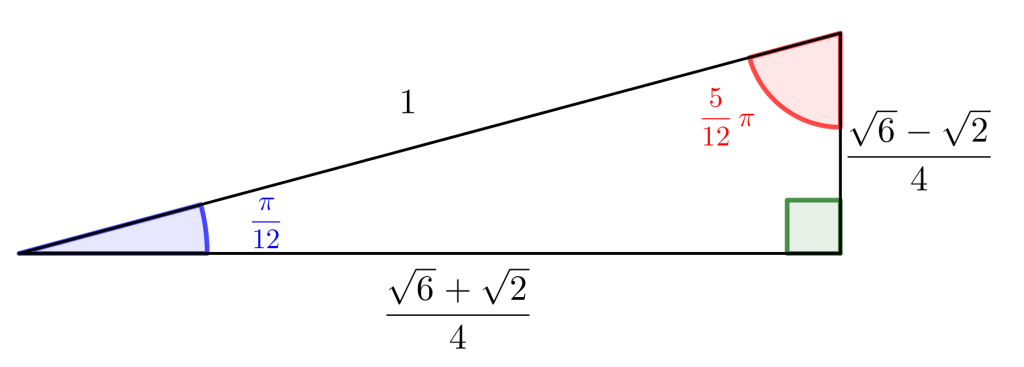

受験に数学が必要な人は、この新しい二つの値も有名角として必ず覚えておこう。
加法定理を利用した三角関数の求め方
加法定理を利用した問題に\(\small{ \ \sin\alpha \ }\)と\(\small{ \ \cos\beta \ }\)などの二つの角の三角関数が与えらているときに\(\small{ \ \sin\left(\alpha+\beta\right) \ }\)の値を求める問題がある。
\(\small{ \ \sin\left(\alpha+\beta\right)=\sin\alpha\cos\beta+\cos\alpha\sin\beta \ }\)だから\(\small{ \ \sin\left(\alpha+\beta\right) \ }\)の値を求めるためには、与えられた\(\small{ \ \sin\alpha \ }\)と\(\small{ \ \cos\beta \ }\)から、\(\small{ \ \cos\alpha \ }\)や\(\small{ \ \sin\beta \ }\)を求める必要がある。
そのときに利用するのが三角関数の相互関係式で、忘れがちだけど\(\small{ \ \sin\theta \ }\)から\(\small{ \ \cos\theta \ }\)とかを求めるために使う重要な式だからね。
\(\small{ \ \tan\theta=\displaystyle\frac{\sin\theta}{\cos\theta} \ }\)
\(\small{ \ 1+\tan^2\theta=\displaystyle\frac{1}{\cos^2\theta} \ }\)
\(\small{ \ 1+\displaystyle\frac{1}{\tan^2\theta}=\displaystyle\frac{1}{\sin^2\theta} \ }\)
\(\small{ \ \alpha \ }\)が鋭角、\(\small{ \ \beta \ }\)が鈍角のとき、次の値を求めよ。
(1)\(\small{ \ \sin\alpha=\displaystyle\frac{1}{3} \ }\)、\(\small{ \ \cos\beta=-\displaystyle\frac{2}{5} \ }\)のとき、\(\small{ \ \sin\left(\alpha+\beta\right) \ }\)
(2)\(\small{ \ \tan\alpha=1 \ }\)、\(\small{ \ \tan\beta=-2 \ }\)のとき、\(\small{ \ \tan\left(\alpha-\beta\right) \ }\)
(1)\(\small{ \ \sin\alpha=\displaystyle\frac{1}{3} \ }\)より
\(\small{ \ \cos^2\alpha=1-\left(\displaystyle\frac{1}{3}\right)^2=\displaystyle\frac{8}{9} \ }\)
\(\small{ \ \alpha \ }\)は鋭角より\(\small{ \ \cos\alpha=\displaystyle\frac{2\sqrt{2}}{3} \ }\)
\(\small{ \ \cos\beta=-\displaystyle\frac{2}{5} \ }\)より
\(\small{ \ \sin^2\beta=1-\left(-\displaystyle\frac{2}{5}\right)^2=\displaystyle\frac{21}{25} \ }\)
\(\small{ \ \beta \ }\)は鈍角より\(\small{ \ \sin\beta=\displaystyle\frac{\sqrt{21}}{5} \ }\)
加法定理を利用して
\(\small{\begin{eqnarray} \ \sin\left(\alpha+\beta\right)&=&\sin\alpha\cos\beta+\cos\alpha\sin\beta\\
&=&\displaystyle\frac{1}{3}\cdot\left(-\displaystyle\frac{2}{5}\right)+\displaystyle\frac{2\sqrt{2}}{3}\cdot\displaystyle\frac{\sqrt{21}}{5}\\
&=&\displaystyle\frac{2\left(\sqrt{42}-1\right)}{15} \ \end{eqnarray}}\)
(2)加法定理を利用して
\(\small{\begin{eqnarray} \ \tan\left(\alpha-\beta\right)&=&\displaystyle\frac{\tan\alpha-\tan\beta}{1+\tan\alpha\tan\beta}\\
&=&\displaystyle\frac{1-(-2)}{1+1\times(-2)}\\
&=&-3 \ \end{eqnarray}}\)

Point 加法定理と15度と75度の三角比
①加法定理は確実に覚える
⓶15度の整数倍の三角関数の値は加法定理から求める

